In preparation for an exciting Short Term, Nate looked into showing the path of a particle as it moves along a constrained path. First he considered the case of a bead down a wire, then a rolling ball down a track. Later he considered the behavior of a loosely bound particle (e.g. a zip line). When considering the forces on a curved track, it is important to understand just how curved the track is. Mathematically, we can look at tangent circles, also known as osculating circles.

Each of the following links leads to a page with additional examples. The animation on this page is an example of what you will find on the linked page. Each animation runs a different amount of time; to see the page's animations again, click "Refresh" on your web browser.

Bead on a wire	Osculating circles
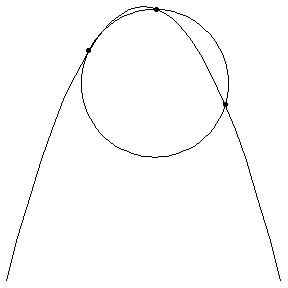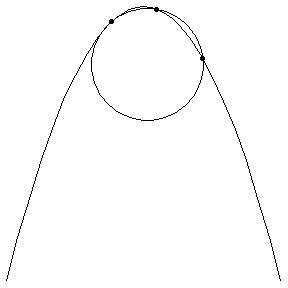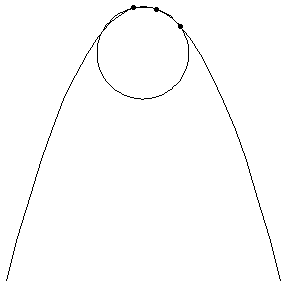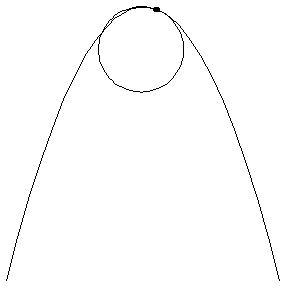Rolling ball	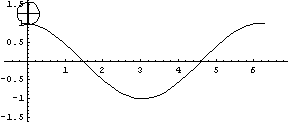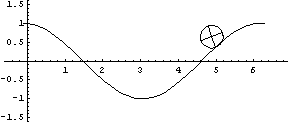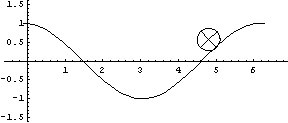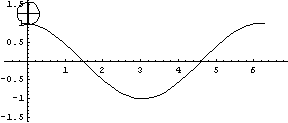Loosely bound particle

To look at the webpage Nate set up, go to http://abacus.bates.edu/~nstambau/.